Currently
This section contains presentations of assignments which ES-Consult have recently finished. The list on the right shows an overview of the examples which have been presented in the course of time.
Presented examples:
Comfort Investigations of Pedestrian Bridges
Modern pedestrian bridges are often slender and light constructions. Structures of this kind are sensitive to the dynamic nature of pedestrian loads. In this connection the notion of comfort refers to the magnitude of accelerations experienced by the users.
ES-Consult has developed a method of calculation which allows realistic assessments of comfort in pedestrian bridges. Additionally, the method can handle application of tuned mass dampers. In the following a short introduction to the method is given, succeeded by an example of an actual application.
The calculation is based on a modal analysis in which the load of pedestrians is applied as a stochastic load. The load consists of parameters such as pacing, harmonic components of footprints and their phaselag, body mass, distribution of pedestrian arrival time, pedestrian traffic intensity and so forth. The parameters are assigned stochastic distributions. By numerical integration the corresponding dynamic response of the bridge is calculated.
A series of these simulations are carried out. On this basis a realistic assessment of the comfort properties of the bridge can be performed.
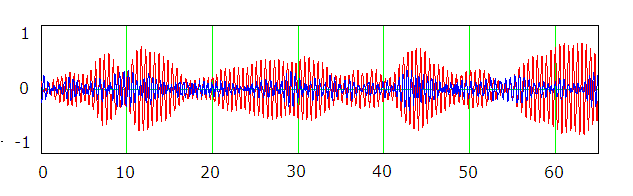
In an atual case a pedestrian bridge with a length of 80m is analysed. The bridge constists of four spans and has a width of four metres. The traffic intensity is assumed to be large, corresponding to an average arrival frequency of three persons from each side. In Figure 1 the principal configuration of the bridge is shown. Figure 2 shows the vertical accelation as a function of time at the center of the third span. The red curve represents the acceleration without application of tuned mass dampers, and the blue curve shows the acceleration with application of tuned mass dampers. As the figure implies, the vibrations of the bridge are subject to a noticeable reduction when tuned mass dampers are applied.
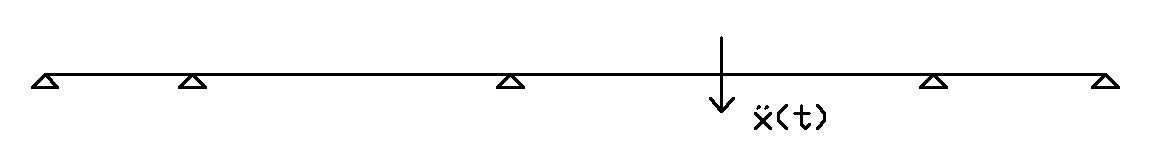
Figure 1. Principal configuration of the bridge.